The square root is among the most fundamental mathematical operations. The square root is a simple-to-understand concept and has numerous applications in various areas. Understanding square roots is the first step before starting various topics in math. There are two main types of square roots in math.
A number's square root is a number that gives the original number when multiplied by itself. Specifically, squares and square roots are exponents. Think of the number nine. The number three gives the result 9 when multiplied by itself. You can write this as 3 x 3 or 32 . You can consider this as a square because the exponent is 2. An example is √n = n1/2, where n is a positive integer.
Calculating the square root of a number
Finding the square root of an integer that forms a perfect square is fairly simple. For instance, 9 is a perfect square since 9 equals 3 * 3. As a result, 3 is 9's square root, and this may be written as √9 = 3. In general, there are four ways to calculate the square root of any number:
Repeated subtraction method.
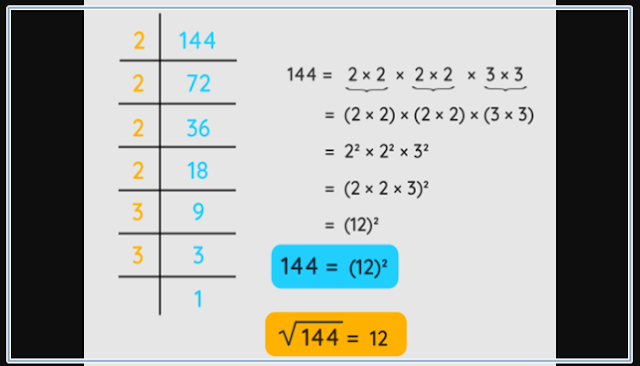
Prime factorization.
Approximation and estimation technique.
Lengthy division technique.
The square root of a decimal number
You can calculate the square root of a decimal number using either the estimation approach or the long division method. For decimal numbers, you can divide the pairings of whole number portions and fractional parts. Then, just like with any other whole number, you divide a long number by a small one.
Calculating the square root of a number with formula
Any number's square root can be calculated using the formula √y = y½. In other words, if the exponent of a number is 1/2, then you must determine the number's square root. Using formula for calculating square root makes the work easy.
The square root of non-perfect numbers
A square number that cannot have its square root expressed as an integer or as a fraction of integers is referred to as a non-perfect or imperfect square number in mathematics. It is possible to get the square root of a non-perfect square number using the long division approach.
Negative square root value
It is possible for a number's square root to be negative. All perfect squares, such as 4, 9, 25, 36, etc., have two square roots, one of which is positive, and the other is negative. The square roots of 4, for example, are -2 and 2. You can see that (-2) (-2) = 4 confirms this. As an example, the square roots of 9 are 3 and -3.
Final thoughts
Algebra and geometry are the two subjects where the square root formula is most frequently utilized. It aids in finding the roots of a quadratic problem. Although it is a simple idea, engineers and mathematicians frequently use it. You should focus on both theory and practice in order to comprehend the fundamentals of math. If you want in-depth information and materials, hire a math teacher or enroll in online math courses.
No comments:
Post a Comment