Children have some amazing grasping power, so it becomes very easy for them to understand different concepts of mathematical that are very helpful for them in the future. From different concepts like addition, subtraction, etc. to the little advanced ones like factors, multiples that can be followed by other advanced topics of mathematics. Here we will discuss the concept of mathematics i.e. Factors of a number.
The factor is a Latin word that means "a maker or a doer". The number that divides the given number without leaving any of the remainders back is the factor of that specific number. To evaluate the factors, it can be done with the help of the multiplication and division method. Just the student needs to be very clear about the concepts related to divisions and multiplication.
Factoring is such a concept that is being widely used in different real-life situations. It will help in dividing a specific thing into equal parts. You can use this concept in dividing the rows and columns and even comparing the prices of the two things. Even different people learn this concept to understand the concept behind the exchange of money, time calculate during the travel. This single concept is used in so many different ways that make it very popular among the people and even very important to understand. So following these simple methods of calculation, the person can easily get all the answers that he requires for his work. Even the clarity in this concept will help the children to learn the prime factorization easily. For more understanding and learning, one can seek the help of experts like Cuemath, It is a leading platform helping students to ace math subject.
There are no such rules that the factor of the number can only be a number, it can also be in the algebraic expressions. The factors of a number can either be a positive or negative number. For example, you take the example of number 6. It factors will be 1, -1, 2, -2, 3, -3, 6, and -6. All these factors are the whole numbers. But when comes to solving a problem mostly the positive numbers are given preference to get the most adequate results.
Here is the list of unbelievable facts to know about the factors of a number. Let's have a look at them.
● The numbers factors are finite: Yes, it is a fact that the factors of a number are limited. For example, we take the number 10, its factors are 1, 2, 5, and 10. So the results are finite and easily approached.
● The factor is always less than or equal to the given number: Whenever we are finding the factors, it is clearly stated that factors are always less than or equal to the given number. Take an example of the number 20, its factors are 1, 2, 4, 5, 10, and 20. All the numbers are less than or equal to the number 20.
● There is an exception in factors of the number: Almost all the numbers have factors but there is an exception to this as well. The numbers 0 and 1 don't have any of the factors, they are the factor of themselves only.
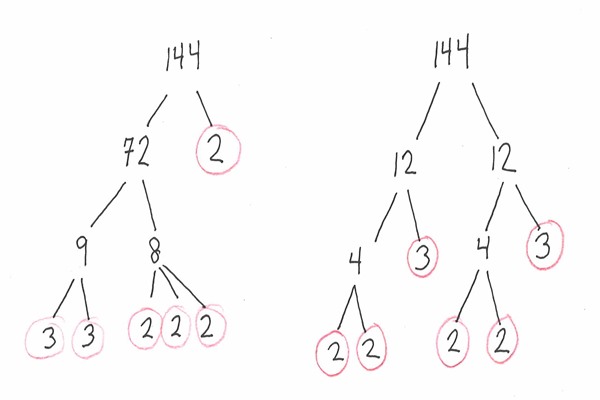
● There are two ways of finding factors: Usually, to calculate the factors of the different numbers, the person takes the help of the two ways i.e. multiplication and division.
To calculate the factors through division methods, the student needs to follow the given procedure. Let’s have a look at them.
1. Find all the numbers that are less than or equal to the given number.
2. After finding the number, divide the two numbers with each other.
3. The divisors that will give the remainder 0 will be the factor of the specific number.
To calculate the factors through the multiplication methods. Here is the list of the procedure to be followed. Let’s have a look at them.
● Write as many products of the given number as possible.
● All the numbers that are involved in the product of the specific numbers are the factors of that number.
No comments:
Post a Comment